On joint discrete universality of the Riemann zeta-function in short intervals
DOI: https://doi.org/10.3846/mma.2023.18884Abstract
In the paper, we prove that the set of discrete shifts of the Riemann zeta-function 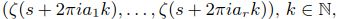
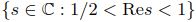

Keywords:
Riemann zeta-function, universality, weak convergenceHow to Cite
Share
License
Copyright (c) 2023 The Author(s). Published by Vilnius Gediminas Technical University.

This work is licensed under a Creative Commons Attribution 4.0 International License.
References
A. Baker. The theory of linear forms in logarithms. In Transcendence Theory: Advances and Applications, pp. 1–27, Boston, 1977. Academic Press.
A. Balčiūnas, V. Garbaliauskienė, V. Lukšienė, R. Macaitienė and A. Rimkevičienė. Joint discrete approximation of analytic functions by Hurwitz zeta-functions. Math. Model. Anal., 27(1):88–100, 2022. https://doi.org/10.3846/mma.2022.15068"> https://doi.org/10.3846/mma.2022.15068
P. Billingsley. Convergence of Probability Measures. Wiley, New York, 1968.
A. Ivič. The Riemann Zeta-Function. Theory and Applications. Dover Publications, Mineola, New York, 2012.
M. Jasas, A. Laurinčikas, M. Stoncelis and D. Šiaučiūnas. Discrete universality of absolutely convergent Dirichlet series. Math. Model. Anal., 27(1):78–87, 2022. https://doi.org/10.3846/mma.2022.15069"> https://doi.org/10.3846/mma.2022.15069
A. Laurinčikas. Limit Theorems for the Riemann Zeta-Function. Kluwer, Dordrecht, 1996. https://doi.org/10.1007/978-94-017-2091-5"> https://doi.org/10.1007/978-94-017-2091-5
A. Laurinčikas. Universality of the Riemann zeta-function in short intervals. J. Number Theory, 204:279–295, 2019. https://doi.org/10.1016/j.jnt.2019.04.006"> https://doi.org/10.1016/j.jnt.2019.04.006
A. Laurinčikas. Discrete universality of the Riemann zeta-function in short intervals. Appl. Anal. Discrete Math., 14(2):382–405, 2020. https://doi.org/10.2298/AADM190704019L"> https://doi.org/10.2298/AADM190704019L
A. Laurinčikas. On joint universality of the Riemann zeta-function. Math. Notes, 110(1-2):210–220, 2021. https://doi.org/10.1134/S0001434621070221"> https://doi.org/10.1134/S0001434621070221
A. Laurinčikas. Joint universality in short intervals with generalized shifts for the Riemann zeta-function. Mathematics, 10(10):art. no. 1652, 2022. https://doi.org/10.3390/math10101652"> https://doi.org/10.3390/math10101652
K. Matsumoto. A survey on the theory of universality for zeta and L-functions. In M. Kaneko, S. Kanemitsu and J. Liu(Eds.), Number Theory: Plowing and Starring Through High Wawe Forms, Proc. 7th China-Japan Semin. (Fukuoka 2013), volume 11 of Number Theory and Appl., pp. 95–144, New Jersey, London, Singapore, Beijing, Shanghai, Hong Kong, Taipei, Chennai, 2015. World Scientific Publishing Co. https://doi.org/10.1142/9789814644938_0004"> https://doi.org/10.1142/9789814644938_0004
S.N. Mergelyan. Uniform approximations to functions of complex variable. Usp. Mat. Nauk., 7(2):31–122, 1952 (in Russian).
H.L. Montgomery. Topics in Multiplicative Number Theory. Lecture Notes Math. Vol. 227, Springer-Verlag, Berlin, 1971. https://doi.org/10.1007/BFb0060851"> https://doi.org/10.1007/BFb0060851
T. Nakamura. The joint universality and the generalized strong recurrence for Dirichlet L-functions. Acta Arith., 138(4):357–362, 2009. https://doi.org/10.4064/aa138-4-6"> https://doi.org/10.4064/aa138-4-6
L . Pańkowski. Joint universality for dependent L-functions. Ramanujan J., 45:181–195, 2018. https://doi.org/10.1007/s11139-017-9886-5"> https://doi.org/10.1007/s11139-017-9886-5
A. Reich. Werteverteilung von Zetafunktionen. Arch. Math., 45:440–451, 1980. https://doi.org/10.1007/BF01224983"> https://doi.org/10.1007/BF01224983
J. Steuding. Value-Distribution of L-Functions. Lecture Notes Math. vol. 1877, Springer, Berlin, Heidelberg, 2007. https://doi.org/10.5565/PUBLMAT_PJTN05_12"> https://doi.org/10.5565/PUBLMAT_PJTN05_12
S.M. Voronin. Theorem on the “universality” of the Riemann zeta-function. Izv. Akad. Nauk SSSR, Ser. Matem., 39:475–486, 1975 (in Russian).
View article in other formats
Published
Issue
Section
Copyright
Copyright (c) 2023 The Author(s). Published by Vilnius Gediminas Technical University.
License

This work is licensed under a Creative Commons Attribution 4.0 International License.

