Regularizing effect in singular semilinear problems
DOI: https://doi.org/10.3846/mma.2023.18616Abstract
We analyze how different relations in the lower order terms lead to the same regularizing effect on singular problems whose model is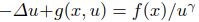 
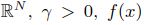
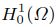
in Ω, u = 0 on ∂Ω, where Ω is a bounded open set of 
is a nonnegative function in L1(Ω) and g(x,s) is a Carathéodory function. In a framework where no 
solution is expected, we prove its existence (regularizing effect) whenever the datum f interacts conveniently either with the boundary of the domain or with the lower order term.
Keywords:
nonlinear elliptic equations, singular problem, regularizing effectHow to Cite
Share
License
Copyright (c) 2023 The Author(s). Published by Vilnius Gediminas Technical University.
This work is licensed under a Creative Commons Attribution 4.0 International License.
References
D. Arcoya and L. Boccardo. Regularizing effect of the interplay between coefficients in some elliptic equations. J. Funct. Anal., 268(5):1153–1166, 2015. https://doi.org/10.1016/j.jfa.2014.11.011"> https://doi.org/10.1016/j.jfa.2014.11.011
D. Arcoya and L. Moreno-Mérida. Multiplicity of solutions for a Dirichlet problem with a strongly singular nonlinearity. Nonlinear Anal., 95:281–291, 2014. https://doi.org/10.1016/j.na.2013.09.002"> https://doi.org/10.1016/j.na.2013.09.002
L. Boccardo, S. Buccheri and C.A. dos Santos. An elliptic system with singular nonlinearities: existence via non variational arguments. J. Math. Anal. Appl., 516(1):Paper No. 126490, 21 pp., 2022. https://doi.org/10.1016/j.jmaa.2022.126490"> https://doi.org/10.1016/j.jmaa.2022.126490
L. Boccardo and L. Orsina. Semilinear elliptic equations with singular nonlinearities. Calc. Var. Partial Differential Equations, 37(3-4):363–380, 2010. https://doi.org/10.1007/s00526-009-0266-x"> https://doi.org/10.1007/s00526-009-0266-x
J. Carmona, T. Leonori, S. López-Martínez and P.J. Martínez-Aparicio. Quasilinear elliptic problems with singular and homogeneous lower order terms. Nonlinear Anal., 179:105–130, 2019. https://doi.org/10.1016/j.na.2018.08.002"> https://doi.org/10.1016/j.na.2018.08.002
J. Carmona and P.J. Martínez-Aparicio. A singular semilinear elliptic equation with a variable exponent. Adv. Nonlinear Stud., 16(3):491–498, 2016. https://doi.org/10.1515/ans-2015-5039"> https://doi.org/10.1515/ans-2015-5039
M.G. Crandall, P.H. Rabinowitz and L. Tartar. On a Dirichlet problem with a singular nonlinearity. Comm. Partial Differential Equations, 2(2):193–222, 1977. https://doi.org/10.1080/03605307708820029"> https://doi.org/10.1080/03605307708820029
W. Fulks and J.S. Maybee. A singular non-linear equation. Osaka Math. J., 12:1–19, 1960.
D. Giachetti, P.J. Martínez-Aparicio and F. Murat. A semilinear elliptic equation with a mild singularity at u = 0: existence and homogenization. J. Math. Pures Appl. (9), 107(1):41–77, 2017. https://doi.org//10.1016/j.matpur.2016.04.007"> https://doi.org//10.1016/j.matpur.2016.04.007
D. Giachetti, P.J. Martínez-Aparicio and F. Murat. Definition, existence, stability and uniqueness of the solution to a semilinear elliptic problem with a strong singularity at u = 0. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5), 18(4):1395–1442, 2018. https://doi.org/10.48550/arXiv.1606.07267"> https://doi.org/10.48550/arXiv.1606.07267
A.V. Lair and A.W. Shaker. Entire solution of a singular semilinear elliptic problem. J. Math. Anal. Appl., 200(2):498–505, 1996. https://doi.org/10.1006/jmaa.1996.0218"> https://doi.org/10.1006/jmaa.1996.0218
A.V. Lair and A.W. Shaker. Classical and weak solutions of a singular semilinear elliptic problem. J. Math. Anal. Appl., 211(2):371–385, 1997. https://doi.org/10.1006/jmaa.1997.5470"> https://doi.org/10.1006/jmaa.1997.5470
A.C. Lazer and P.J. McKenna. On a singular nonlinear elliptic boundary-value problem. Proc. Amer. Math. Soc., 111(3):721–730, 1991. https://doi.org/10.1090/S0002-9939-1991-1037213-9"> https://doi.org/10.1090/S0002-9939-1991-1037213-9
F. Oliva and F. Petitta. On singular elliptic equations with measure sources. ESAIM Control Optim. Calc. Var., 22(1):289–308, 2016. https://doi.org/10.1051/cocv/2015004"> https://doi.org/10.1051/cocv/2015004
F. Oliva and F. Petitta. Finite and infinite energy solutions of singular elliptic problems: existence and uniqueness. J. Differential Equations, 264(1):311–340, 2018. https://doi.org/10.1016/j.jde.2017.09.008"> https://doi.org/10.1016/j.jde.2017.09.008
G. Stampacchia. Le problème de Dirichlet pour les équations elliptiques du second ordre à coefficients discontinus. Ann. Inst. Fourier (Grenoble), 15(fasc. 1):189–258, 1965.
C.A. Stuart. Existence and approximation of solutions of non-linear elliptic equations. Math. Z., 147(1):53–63, 1976. https://doi.org/10.1007/BF01214274"> https://doi.org/10.1007/BF01214274
Z. Zhang and J. Cheng. Existence and optimal estimates of solutions for singular nonlinear Dirichlet problems. Nonlinear Anal., 57(3):473–484, 2004. https://doi.org/10.1016/j.na.2004.02.025"> https://doi.org/10.1016/j.na.2004.02.025
View article in other formats
CrossMark check
Published
Issue
Section
Copyright
Copyright (c) 2023 The Author(s). Published by Vilnius Gediminas Technical University.
License
This work is licensed under a Creative Commons Attribution 4.0 International License.